Meccano construct a 17-gon
I recently read an interesting manuscript: Gerard ’t Hooft, Meccano Math I (2006)
I will summarize the key bits here and then present a bit of a challenge.
Meccano bars are metal strips with holes in them at integer lengths. A bar of length \$n\$ has \$n+1\$ holes evenly spaced along it. Two bars may be attached at a two of their holes to form a joint.
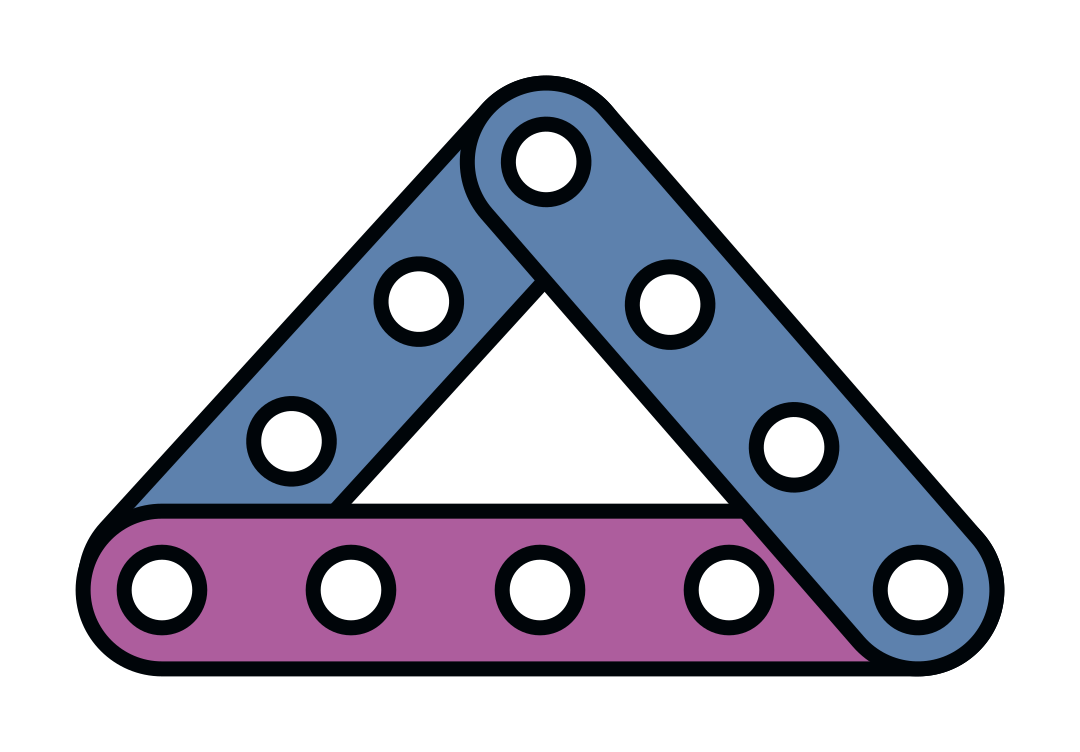
A configuration is rigid when there is exactly one way to connect the given bars at the specified holes. For example all triangles are rigid:
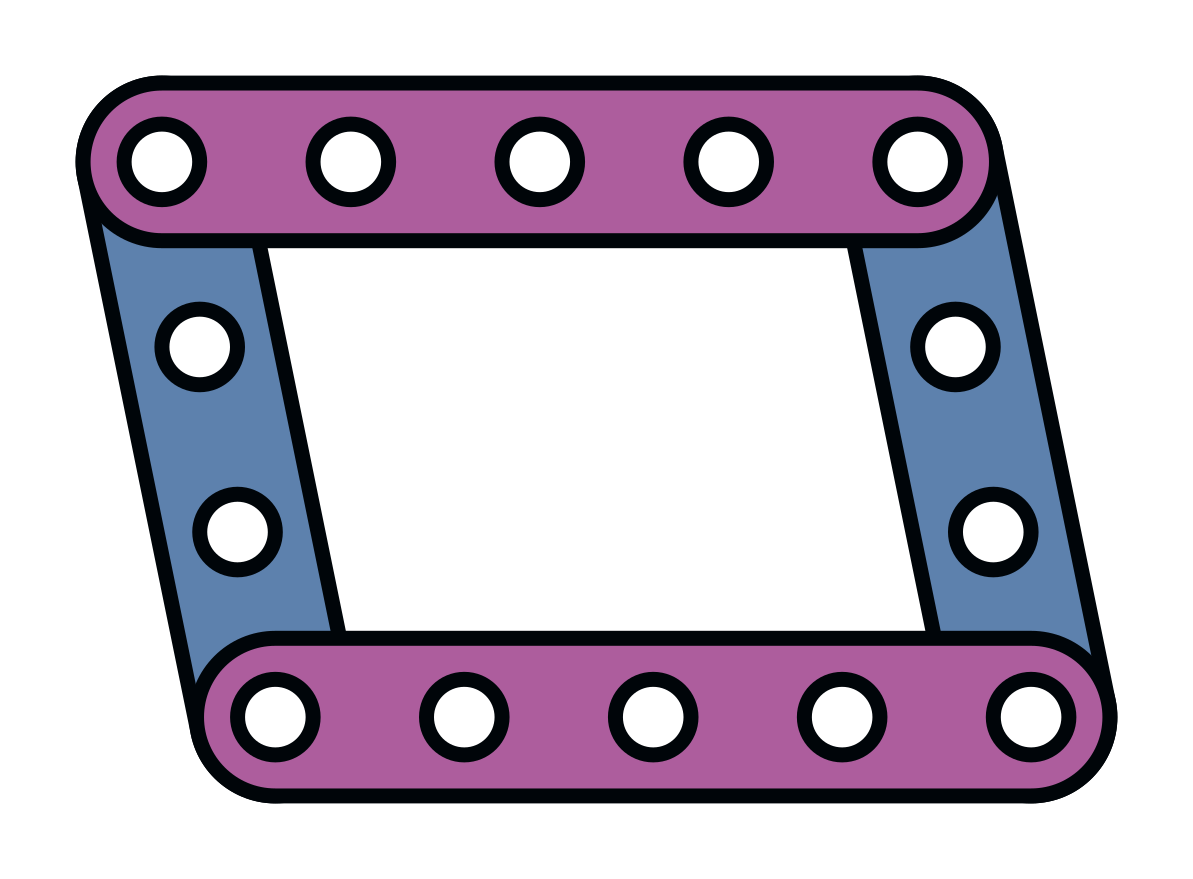
However quadrilaterals are nearly universally non-rigid:
As the joints share a degree of freedom. All configurations with a degree of freedom are not rigid, however we don't necessarily require thatnot all configuration zero degrees of freedom beconfigurations are rigid. For example
EXAMPLE HERE
Task
Your task is to produce a set of bars, along with their lengths and which holes are attached such that it forms a rigid construction, and 17 of the Meccano bars in your solution form the edges of a regular 17-gon.
Scoring
Your score will be the number of Meccano bars used in your solution, with ties being broken by the sum of their lengths. Your goal is to minimize your score.